Week 9

• Problem (posted October 29th)

  In triangle $\Delta ABC$ we denote as usual $AB=c, AC=b, BC=a$. Let $D$ be the point on the side $BC$ such that $AD$ is the bisector of $\angle BAC$. Let $E$ be point symmetric to $D$ with respect to the midpoint $M$ of $BC$, and let $F$ be the point inside the segment $BC$, such that $\angle BAF =\angle EAC$. Show that $$ \frac{BF}{FC}=\frac{c^3}{b^3} \,. $$

• Solution (posted November 5th)

  Problem 4 of the 30th Spanish Mathematical Olympiad, First Round, 1993 , which appeared in Crux Mathematicorum [1998: 69]. We present the solution by Toshio Seimiya, which appeared at [1999: 201-203].

  Note: An extended video solution to this problem is also available and includes an alternative solution involving the Law of Sines.

  Let $T$ be the point on $AF$ so that $BT \parallel AC$ and let $S$ be the point on $AE$ so that $CS \parallel AB$.

  

  Then \begin{align} \angle ABT&+\angle BAC=180^\circ \cr \angle ACS&+\angle BAC=180^\circ \,. \end{align} Then, $\angle ABT = \angle ACS$ and $\angle BAT =\angle CAS$ showing that $\Delta BAT \sim \Delta CAS$. This gives \begin{equation}\tag{1} \frac{AT}{AS}=\frac{BT}{CS}=\frac{c}{b} \,. \end{equation}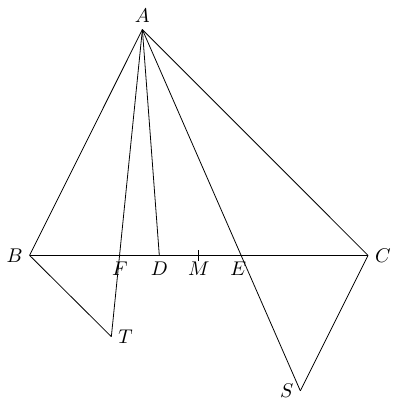

  As $BT \parallel AC$ we get $\Delta BFT \sim \Delta CFA$ and hence \begin{equation}\tag{2} \frac{BF}{FC}=\frac{FT}{AF}=\frac{BT}{b} \,. \end{equation}

  Similarly, $CS \parallel AB$ implies $\Delta ABE \sim \Delta CSE$ and hence \begin{equation}\tag{3} \frac{BE}{CE}=\frac{AE}{SE}=\frac{c}{CS} \,. \end{equation}

  Since $E$ is the symmetric of $D$ with respect to $M$ we have $EC=BD$ and $BE=CD$, and hence $$ \frac{BE}{EC}=\frac{DC}{DB} \,. $$

  Finally, since $AD$ is the bisector of $\angle BAC$, the Bisector Theorem gives \begin{equation}\tag{4} \frac{DC}{DB}=\frac{b}{c} \,. \end{equation}

  Combining (3) and (4) we get $$ \frac{c}{CS}=\frac{b}{c} \Rightarrow CS=\frac{c^2}{b} \,. $$ Therefore, by (1) we get $$ BT=\frac{c^3}{b^2} \,. $$

  Finally, (2) gives $$ \frac{BF}{FC}=\frac{BT}{b}=\frac{c^3}{b^3} \,. $$ ∎

Week 8

• Problem (posted October 22nd)

  Let $a_1,a_2,..., a_n, b_1, b_2,...b_n$ be $2n$ real numbers such that $a_1,a_2,..., a_n$ are distinct. If there exists a real number $\alpha$ such that for each $1 \leq j \leq n$ we have $$ (a_j+b_1)\cdot(a_j+b_2) \cdot \dots \cdot (a_j+b_n) =\alpha \,, $$ show that there exists a number $\beta$ such that for each $1 \leq k\leq n$ we have $$ (a_1+b_k)\cdot(a_2+b_k) \cdot \dots \cdot (a_n+b_k) =\beta \,. $$

• Solution (posted October 29th)

  Problem 2 of the Second paper, Sixth Irish Mathematical Olympiad, which appeared in Crux Mathematicorum [1995: 152]. We present the solution by Michael Selby, which appeared at [1997: 141-142].

  Note: A video solution to this problem is also available.

  Define $$ P(X)=(X+b_1)\cdot(X+b_2)\cdot \dots \cdot (X+b_n) -\alpha\,. $$ Then, $P(a_1)=P(a_2)=...=P(a_n)=0$. Therefore, since $a_1,a_2,...,a_n$ are dintinct, by the Factor Theorem we have $$ P(X)=(X-a_1)\cdot(X-a_2) \cdot \dots \cdot (X-a_n) \,. $$ This gives \begin{equation}\tag{1} (X+b_1)\cdot(X+b_2)\cdot \dots \cdot (X+b_n) -\alpha=(X-a_1)\cdot(X-a_2) \cdot \dots \cdot (X-a_n) \,. \end{equation} Now, for each $1 \leq k \leq n$, setting $X=-b_k$ in (1) gives $$ 0-\alpha=(-1)^{n} (a_1+b_k)\cdot(a_2+b_k) \cdot \dots \cdot (a_n+b_k) \,. $$ Therefore, for all $1 \leq k \leq n$ we have $$ (a_1+b_k)\cdot(a_2+b_k) \cdot \dots \cdot (a_n+b_k) = (-1)^{n+1} \alpha \,. $$ Setting $\beta=(-1)^{n+1} \alpha$ gives the claim.

Week 7

• Problem (posted October 15th)

  An equilateral triangle of side length $6$ is divided into $36$ small equilateral triangles of side length $1$. We construct pieces of type $A$ and type $B$ by joining triangles of side length $1$, as in the diagram below. By using $m$ pieces of type $A$ and n pieces of type $B$, with no overlapping and no gaps, like the pieces of a jigsaw puzzle, we can construct the original large triangle. Determine all possible values of $m$.

  

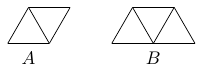
• Solution (posted October 22nd)

  Problem 2 Olimpiada Nacional Escolar de Matematica 2009, Level 2, which appeared in Crux Mathematicorum at [2010: 373]. We present the solution by Chip Curtis, which appeared at [2011: 425-426], modified by the editor.

  Note: A video solution to this problem is also available.

  Since each piece of type $A$ covers two triangles, and each piece of type B covers three triangles, we have $$ 2m+ 3n = 36 \,. $$ This yields $$ m \in \{ 0, 3, 6, 9, 12, 15, 18 \} \,. $$ We prove that $m = 0, 3, 6, 9$ are possible, while $m = 12, 15, 18$ are impossible.

  The following pictures show that $m=0,3,6,9$ are possible.

  

  

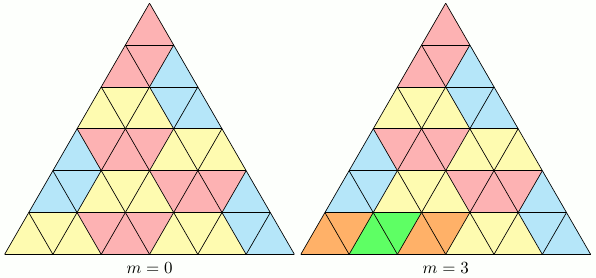
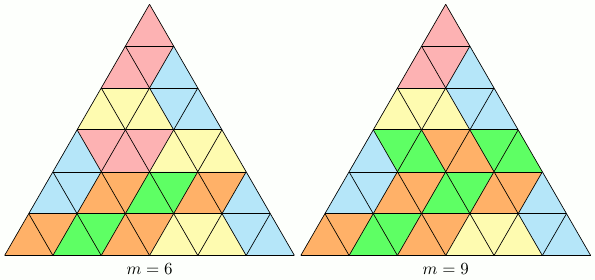
  To see that $m = 12, 15, 18$ are impossible, color the small triangles with two colors alternately.

  

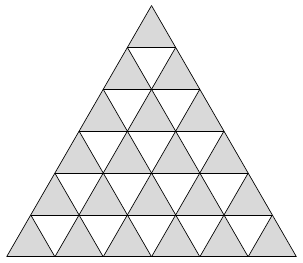
  Note that any piece of type $A$ covers exactly one white and one gray triangle. Any piece of type $B$ covers either two white and one gray triangles, or one white and two gray triangle.

  Since there are 6 more gray triangle than white triangles, we need to use at least $6$ pieces of type $B$, that is $n \geq 6$. Therefore $$ m=\frac{36-3n}{2} \leq \frac{36-18}{2} =9 \,. $$

  This shows that $$ m \in \{ 0, 3, 6, 9 \} \,. $$

Week 6

• Problem (posted October 8th)

  Find all the functions $f : \mathbb R \to \mathbb R$ with the property that for all $x,y \in \mathbb R$ we have \begin{equation}\tag{1} f \left(xf(x)+f(y) \right)= \left(f(x) \right)^2+y .\end{equation}

• Solution (posted October 15th)
  Note: A video solution to this problem is also available.

  Problem 1 of the 17th Balkan Mathematical Olympiad , which appeared in Crux Mathematicorum [2004: 84]. We present the solution by José Luis Díaz-Barrero, which appeared at [2005: 523-524].

  It is easy to see that the function $f(x)=x$ for all $x \in \mathbb{R}$ and the function $f(x)=-x$ for all $x \in \mathbb{R}$ satisfy (1). We show that these are the only solutions.

  Let $a=f(0)$. Setting $x=0$ in (1) we get \begin{equation}\tag{2} f(f(y))=y+a^2 \,. \end{equation} Pick $b=f(-a^2)$. Then $f(b)=f(f(-a^2))=-a^2+a^2=0$.

  Setting $x=b$ in (1) we get \begin{equation}\tag{3} f(f(y))=y \,. \end{equation} By combining (2) and (3) we get $a=0$, and hence $f(0)=0$. Setting $y=0$ in (1) we get \begin{equation}\tag{4} f(xf(x))=\left( f(x) \right)^2 \,. \end{equation}

  Now, let $t \in \mathbb R$ be arbitrary. Setting $x=f(t)$ in (4), and using the fact that by (3) we have $f(x)=t$ we get \begin{equation}\tag{5} f(tf(t))=\left( f(f(t)) \right)^2=t^2 \,. \end{equation}

  Therefore, for all $x \in \mathbb R$ we have by (4) and (5) \begin{equation}\tag{6} x^2=f(xf(x))=(f(x))^2 \,. \end{equation}

  This gives that $f(x)=\pm x$ for each $x \in \mathbb{R}$. Therefore, for each $x \in \mathbb{R}$ we have either $f(x)=x$ or $f(x)=-x$. To complete the solution we need to show that the sign is the same for all $x$.

  Assume by contradiction that there exist non-zero numbers $\alpha, \beta$ such that $f(\alpha)=\alpha$ and $f(\beta)=-\beta$. Then, setting $x=\alpha, y=\beta$ in (1) we get $$ f \left(\alpha^2-\beta \right)= \alpha^2+\beta \,, $$ and hence, by (6) $$ \left(\alpha^2-\beta \right)^2=\left(\alpha^2+\beta \right)^2 \,. $$ Expanding, we get $\alpha^2 \beta=0$ which contradicts the fact that $\alpha, \beta$ are non-zero.

  Since we got a contradiction, our assumption was wrong. Therefore, the only solutions are the function $f(x)=x$ for all $x \in \mathbb{R}$ and the function $f(x)=-x$ for all $x\in \mathbb{R}$.

  Editor's note: This problem is similar to the 2014 COMC Problem of the Week 4, see https://comc.math.ca/2014/potw.html

Week 5

• Problem (posted October 1st)

  Find all the triplets $(x,y,z)$ consisting of positive integers, which satisfy the equation $$ \frac{1}{x}+\frac{2}{y}-\frac{3}{z}=1 $$

• Solution (posted October 8th)

  Problem 3 of the 26th Belgian Mathematical Olympiad , which appeared in Crux Mathematicorum [2004: 268]. We present the solution by Pierre Bornsztein, which appeared at [2006: 153].

  Note: A video solution to this problem is also available.

  Let $(x,y,z)$ be any solution to the equation. Then \begin{equation}\tag{1} yz+2xz=3xy+xyz \,. \end{equation} If $x \geq 3$ and $y \geq 3$ then $3(x-1)\geq2x$ and hence $$ 3xy+xyz> xyz = yz +(x-1)yz \geq yz+3(x-1)z \geq yz+2xz \,, $$ which contradicts (1). Therefore, $x <3$ or $y<3$.

  • First suppose that $y \geq 3$. Then $x=1$ or $x=2$.

    If $x=2$ then $$ yz+4z=6y+2yz \Rightarrow yz+6y-4z=0 \Rightarrow (y-4)(z+6)=-24 \,. $$ Since $y-4 \geq -1$ and $z+6 \geq 7$ we get $y-4=-1$ and $z+6=24$ giving the solution $$ (x,y,z)=(2,3,18) \,. $$ It is easy to check that this is a solution.

    If $x=1$ then $$ yz+2z=3y+yz \Rightarrow 3y= 2z $$ giving the solutions $$ (x,y,z)=(1,2a,3a) \,, $$ for an integer $a \geq 2$ (since $y \geq 3$).

    It is easy to check that all these are solutions.

  • If $y=2$ then the equation becomes $$ 2z+2xz=6x+2xz \Rightarrow z=3x \,. $$ This gives the solutions $$ (x,y,z)=(b,2,3b) \,, $$ for an integer $b \geq 1$. Note that for $b=1$ we get the solution $(1,2,3)$, which corresponds to $a=1$ in the previous paragraph.
  • If $y=1$ then the equation becomes $$ z+2xz=3x+xz\Rightarrow xz-3x+z=0 \Rightarrow (x+1)(z-3)=-3 \,. $$ Since $x+1 \geq 2$ we have $x+1=3, z-3=-1$, giving the solution $$ (x,y,z)=(2,1,2) \,. $$ Conversely, the triple $(2,1,2)$ is a solution.

    Therefore, the solutions are all the triples of the form $(1, 2a, 3a), (a, 2, 3a)$ for positive integer $a$, together with $(2,1,2)$ and $ (2,3,18)$.

Week 4

• Problem (posted September 24th)

  If $$\left(x+\sqrt{x^2+1}\right)\left(y+\sqrt{y^2+1}\right)=1$$ show that $x+y=0$.

• Solution (posted October 1st)

  Problem 2 of the 31st Spanish Mathematical Olympiad, First Round, which appeared in Crux Mathematicorum [1998: 453]. We present the solution by Mohammed Aassila, which appeared at [2000: 142], modified by the editor.

  Note: A video solution to this problem is also available.

  Set $z=x+\sqrt{x^2+1}$. Then, $z>0$ and $$ \frac{1}{z}=\frac{\sqrt{x^2+1}-x}{x^2+1-x^2}=\sqrt{x^2+1}+x-2x=z-2x \,, $$ giving $$ x=\frac{z^2-1}{2z} \,. $$ Then, \begin{align} z\left(y+\sqrt{y^2+1}\right)&=1 \qquad \Rightarrow \cr y+\sqrt{y^2+1}&=\frac{1}{z} \qquad \Rightarrow \cr z=\frac{\sqrt{y^2+1}-y}{y^2+1-y^2}&=\sqrt{y^2+1}+y-2y=\frac{1}{z}-2y \Rightarrow \cr y&=\frac{1-z^2}{2z} \,. \end{align} Hence $$ x+y= \frac{z^2-1}{2z} + \frac{1-z^2}{2z} =0 \,. $$

  ∎

  Editor's note: If you are familiar with logarithms and inverse hyperbolic functions, you will probably like Michel Battaille's solution: Taking logarithms and using $\sinh^{-1}(x)=\ln\left(x+\sqrt{x^2+1}\right)$ the equation becomes $$ \sinh^{-1}(x)+\sinh^{-1}(y) =0 \,. $$ Since $\sinh^{-1}(x)$ is an odd function, and one-to-one, we get $$ \sinh^{-1}(x)=\sinh^{-1}(-y) \Rightarrow x=-y \,. $$

Week 3

• Problem (posted September 17th)

  Define the sequence $a_1, a_2 , a_3 , \dots$ as follows

  $a_1$	$=a_2=1003 $
  $a_{n+1}$	$=a_n-a_{n-1} \qquad$ for all $n \geq 2 \,.$

  Calculate the sum of the first 2006 terms in the sequence.

• Solution (posted September 24th)

  Problem 3 of the Mathematical Olympiad of the Asociación Venezolana de Competencias Matemáticas, 2006, which appeared in Crux Mathematicorum [2009: 380]. We present the solution by Oliver Geupel, which appeared at [2011: 278].

  Note: A video solution to this problem is also available.

  We have

  $a_3$	=$a_2-a_1=0$
  $a_4$	=$a_3-a_2=-1003$
  $a_5$	=$a_4-a_3=-1003$
  $a_6$	=$a_5-a_4=0 $
  $a_7$	=$a_6-a_5=1003=a_1$
  $a_8$	=$a_7-a_6=1003=a_2$

  It follows from here that the sequence is periodic, with period $6$. Moreover, the sum of 6 consecutive terms is zero.

  Therefore, as $2004=6 \cdot 334$ we get

  $\displaystyle\sum_{k=1}^{2006}a_k$	$\displaystyle =a_1+a_2 + \sum_{j=0}^{333} \left( a_{6j+3}+a_{6j+4}+a_{6j+5}+a_{6j+6}+a_{6j+7}+a_{6j+8} \right)$
  	$=a_1+a_2$
  	$=2006 \,.$ ∎

Week 2

• Problem (posted September 10th)

  How many subsets with three elements can be formed from the set $\{ 1 , 2 , 3, \dots , 20 \}$ such that $4$ is a factor of the product of the three numbers in the subset?

• Solution (posted September 17th)

  Problem 1 of the Icelandic Mathematical Contest 2004-05, which appeared in Crux Mathematicorum [2008: 285]. We present the solution by John G. McLoughlin which appeared at [2009: 386].

  Note: A video solution to this problem is also available.

  There are $\binom{20}{3}=1140$ subsets of three elements in total. We count how many do not meet the requirements.

  We first note that a subset meets the requirements excepting in two cases:

  • all elements are odd.
  • two elements are odd, and the third is even but not a multiple of $4$.

  In the first case, there are $\binom{10}{3}=120$ subsets consisting of only odd numbers.

  In the second case, there are $\binom{10}{2}\binom{5}{1}=225$ subsets consisting of two odd numbers and one even number not divisible by $4$.

  Therefore, in total there are $$ 1140-120-225=795 $$ such subsets. ∎

Week 1

• Problem (posted September 3rd)

  We give two entry level problems this week. Give them a try. Look for the source and the solution next week!

  Problem A
  Let $x$ be an integer of the form $$ x=\underbrace{111\dots11}_{n \mbox{ times }} $$ Show that, if $x$ is prime then $n$ is prime.

  Problem B
  Given a rectangle with sides $a$ and $b$, with $a>b$, fold it along the diagonal. Determine the area of the overlapping triangle (the shaded area in the picture).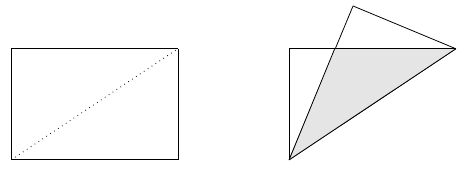

  

• Solution (posted September 10th)

  Problem A

  Problem 7 of the Chilean Mathematical Olympiads 1994-95, which appeared in Crux Mathematicorum [2001: 170]. We present the solution by Christopher J. Bradley that appeared at [2003: 294].

  Note: A video solution to this problem is also available.

  Note that $$ 9x=\underbrace{999\dots 99}_{n \mbox{ times }}=10^n-1 \,. $$ Therefore, $$ x=\frac{10^n-1}{9} \,. $$ Now, if we assume by contradiction that $n$ is composite, $n=n_1n_2$ for some $n_1, n_2 >1$. Then $$ x=\frac{10^n-1}{9}=\frac{10^{n_1}-1}{9} \left(1+10^{n_1}+10^{2n_1}+\dots+10^{n_1(n_2-1)} \right)\,. $$ As $\frac{10^{n_1}-1}{9}$ is an integer greater than $1$ and $1+10^{n_1}+10^{2n_1}+\dots+10^{n_1(n_2-1)}>1$ we get that $x$ is composite, a contradiction.

  Since, we got a contradiction, our assumption that $n$ is composite is wrong.

  Therefore, $n$ is not composite and $n\neq 1$ (since $x=1$ is not prime), which proves that $n$ is prime. ∎

  Editor's note: Primes of this form are sometimes called "repunit primes". So far 5 repunit primes are known, namely the values of $x$ for $n \in \{ 2, 19, 23, 317, 1031 \}$. The values of $x$ for $n \in \{ 49081, 86453, 109297, 270343\}$ are also believed to be prime.

  Problem B

  Problem 1 of the XV Gara Nazionale di Matematica 1999, which appeared in Crux Mathematicorum [2002: 481] . We present the solution by Bob Serkey that appeared at [2005: 37].

  Note: A video solution to this problem is also available.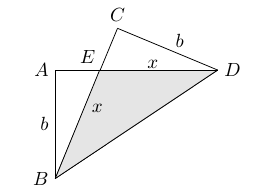

  

  Let $E$ be the intersection of $BC$ and $AD$, and let $x=BE$.

  Note that the right triangles $\Delta AEB$ and $\Delta CED$ have $CD=AB$ and $\angle AEB = \angle CED$, and thus are congruent. Therefore, we have $$ DE=BE=x \,. $$ We therefore also have $$ AE=CE=a-x \,. $$ By Pythagoras theorem we have $$ (a-x)^2+b^2=x^2 \, \Rightarrow \, x=\frac{a^2+b^2}{2a} \,. $$ In the triangle $\Delta BED$, $DC=b$ is the altitude to the base $BE=x$. Therefore $$ \mbox{Area} (BED)=\frac{bx}{2}= \frac{b}{4a} \left(a^2+b^2 \right) \,. $$

  ∎