Week 9

• Problem (posted October 31st)

  The area of a trapezoid is 2 cm$^2$ and the sum of its diagonal is $4$ cm. Find the altitude of the trapezoid.

• Solution (posted November 7th)

  This is problem 4 of the Georgian Math Olympiad 1997, Grade X, which appeared in Crux Mathematicorum in [2000:193]. We give the solution of Amengual Covas that appeared in Crux Mathematicorum in [2002:204-205], modified by the editor.

  Let $AD$ be the small base of $ABCD$ and $CD$ the large base.

  

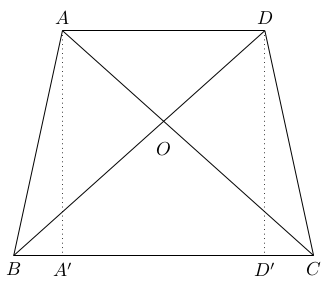
  We first prove that the diagonals are equal and perpendicular at $O$.

  We have \begin{align} \mbox{Area} (ABD) &= \frac{1}{2} BD \cdot AO \cdot \sin(\angle AOB) \cr \mbox{Area} (CBD) &= \frac{1}{2} BD \cdot CO \cdot \sin(\angle COD) \cr 2=\mbox{Area} (ABCD) &= \frac{1}{2} BD \cdot AC \cdot \sin(\angle AOB) \cr \end{align}

  Let us denote $BD=x$. Then $AC=4-x$, and hence $$4= x (4-x) \sin(\angle AOB) \leq x(4-x) =4x-x^2=4-(x-2)^2 \leq 4 \,.$$

  Therefore, all inequalities in the above line are equalities. This implies that \begin{align} 4-(x-2)^2 &= 4 \qquad \mbox{and} \cr x (4-x) \sin(\angle AOB) &= x(4-x). \cr \end{align}

  The first equality gives $x=2$, and therefore $BD=x=2=4-x=AC$. The second equality gives $\angle AOB=90^\circ$ and hence $AC \perp BD$.

  Next, we show that $AB=CD$, that is the trapezoid is isosceles. To see this, drop perpendiculars $AA',DD'$ from $A$ and $D$, respectively, on the side $BC$.

  We have \begin{align} AC &=BD \cr AA' &= DD' \cr \angle AA'C & =\angle DD'B= 90^\circ \cr \end{align}

  This implies that the right triangles $AA'C$ and $DD'B$ are congruent, and hence $A'C=BD'$. From here, we get $$A'B=CD' \,.$$

  Now, the right triangles $AA'B$ and $DD'C$ are congruent, since \begin{align} A'B &= D'C \cr AA' &= DD' \cr \angle AA'B & =\angle DD'C= 90^\circ \cr \end{align} and hence $AB=CD$.

  Since $ABCD$ is an isosceles trapezoid, it follows immediately that $BOC$ is an isosceles right triangle, and hence $\angle OCB=45^\circ$. From here, we get that $AA'=A'C$.

  Finally \begin{align} 2 &= \mbox{area}(ABCD) = \mbox{area}(ABA')+ \mbox{area}(AA'D'D)+ \mbox{area}(CDD') \cr &= \mbox{area}(AA'D'D)+ 2\mbox{area}(CDD') \cr &= AA' \cdot A'D'+ DD' \cdot D'C= AA'\cdot A'C \cr \end{align}

  Since $AA'=A'C$ we get that $A'A^2=2$ and hence $$AA'=\sqrt{2} \,.$$

Week 8

• Problem (posted October 24th)

  An acute triangle $ABC$ is inscribed in a circle with the center at $O$. The bisector of $\angle A$ meets the side $BC$ at $D$. The perpendicular from $D$ to $AO$ meets the side $AC$ at a point $P$ which is interior to $AC$. Show that $AB=AP$.

• Solution (posted October 31st)

  This is problem 5 of the 1995 Italian Math Olympiad which appeared in Crux Mathematicorum in [1998:323-324]. We give the solution of Pierre Bornsztein that appeared in Crux Mathematicorum in [2000:79-80].

  

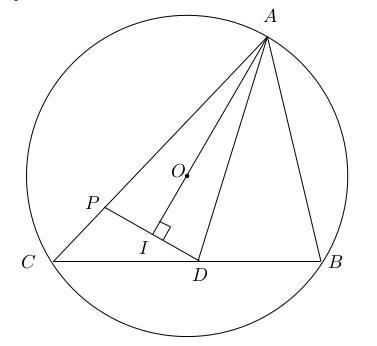
  Since $ABC$ is acute, $O$ is interior to $ABC$. Since $P$ is interior to $AC$, $O$ is interior to $ADC$.

  Let us denote as usual $\angle A= \alpha, \angle B= \beta$ and $\angle C= \gamma$. We have \begin{align} \angle AOC &= 2 \beta \cr AO&=OC \cr \end{align} and therefore, $$\angle CAO = \frac{1}{2} ( 180^\circ -2 \beta) =90^\circ - \beta \,.$$

  This yields $$\angle OAD = \frac{\alpha}{2}-\left(90^\circ - \beta \right)=\frac{\alpha}{2} +\beta -90^\circ \,.$$

  Let us denote by $I$ the intersection between $AO$ and $PD$. Then, the triangle $\Delta AID$ is right at $I$ and hence \begin{align} \angle PDA &=\angle IDA = 90^\circ - \angle OAD \cr &=180^\circ - \frac{\alpha}{2}-\beta \cr &=180^\circ - \angle DAB -\angle DBA =\angle ADB \,.\cr \end{align}

  Therefore, we have \begin{align} \angle PDA &=\angle ADB \cr AD &=AD \cr \angle PAD &= \angle BAD. \cr \end{align}

  This shows that the triangles $\Delta PDA$ and $\Delta BDA$ are congruent, and hence $PA=BA$.

Week 7

• Problem (posted October 17th)

  Do there exist a number $q \in \mathbb N$ and a prime number $p \in \mathbb N$ such that $$3^p+7^p = 2 \cdot 5^q?$$

• Solution (posted October 24th)

  This is problem 4 of the Ukrainian Math Olympiad, 11th Grade, which appeared in Crux Mathematicorum in [2006:217-218]. We give the solution of Michel Bataille that appeared in Crux Mathematicorum in [2007:227-228].

  There is no such pair $(p,q)$. To prove this, we argue by contradiction.

  Assume by contradiction that a pair $(p,q)$ exists. It is straightforward to check that $p=2$ doesn't work. Then, $p$ is odd, and hence $$2 \cdot 5^q=3^p+7^p=10 \cdot (3^{p-1}-3^{p-2}\cdot 7+...-3 \cdot 5^{p-2}+7^{p-1}).$$

  It follows that \begin{align} 3^{p-1}-3^{p-2}\cdot 7+...-3 \cdot 7^{p-2}+7^{p-1}&=5^{q-1} \,.\tag{1}\cr \end{align}

  A fast computation shows that $q=1$ cannot satisfy the initial equation, and hence $5^{q-1} \equiv 0 \pmod{5}$. Now, using $3\equiv -2 \pmod{5}$ and $7\equiv 2 \pmod{5}$, and $p$ odd, in (1) we get $$2^{p-1}+2^{p-1}+...+2^{p-1}=p2^{p-1} \equiv 0 \pmod{5} \,.$$

  This implies that $p=5$ and hence $$2 \cdot 5^2 \cdot 341=3^5+7^5 = 2 \cdot 5^q \,,$$ which is a contradiction.

Week 6

• Problem (posted October 10th)

  This week we look at a problem about two polynomials.

  Let $P(X)$ and $Q(X)$ be two polynomials with integer coefficients. If $\frac{P(n)}{Q(n)}$ is an integer for every integer $n$, prove that there exists a polynomial $S(X)$ with rational coefficients such that $P(X)=Q(X)S(X)$.

• Solution (posted October 17th)

  This is problem 5 of Alberta High School Math Competition 1995, Part B. We present the official solution.

  We divide $P(X)$ by $Q(X)$ and obtain a quotient $S(X)$ and a remainder $R(X)$. Then, we have $$P(X)=Q(X)S(X)+R(X) \,,$$ with $\deg(R(X)) < \deg(Q(X))$. We then have: $$ \begin{align} \frac{P(X)}{Q(X)}&= S(X)+ \frac{R(X)}{Q(X)} \,.\tag{1}\cr \end{align} $$

  Since both $P(X)$ and $Q(X)$ have integer coefficients, $S(X)$ has rational coefficients. To complete the proof we show that $R(X)=0$.

  We can write $$S(X)=\frac{1}{m} T(X) \,,$$ for some integer $m > 0$ and some polynomial with integer coefficients. By combining the hypothesis with (1) we then get that for all integers $n$ we have $$m \frac{R(n)}{Q(n)} = m\frac{P(n)}{Q(n)}-T(n) \in \mathbb Z \,.$$

  Since $\deg(Q(x)) > \deg(R(x))$, for $x$ sufficiently large we have $$\left| m \frac{R(x)}{Q(x)} \right| < 1\,.$$

  Therefore, for all integers $n$ which are sufficiently large we get that $\left| m \frac{R(n)}{Q(n)} \right|=0$ and hence, that $R(n)=0$. This shows that $R$ has infinitely many roots, and thus $R=0$, as claimed.

  Editor note: Note that we cannot conclude that $S(x)$ has integer coefficients. Indeed, if $P(X)=X^2+X$ and $Q(X)=2$ then $\frac{P(n)}{Q(n)}=\frac{n(n+1)}{2}$ is an integer for all integers $n$ but $S(X)=\frac{1}{2}X^2+\frac{1}{2}$.

  Pólya proved that a polynomial $P(X)$ takes integer values at all integers if and only if there exists some $n$ and integers $a_0,..,a_n$ such that $$ \begin{align} P(X)&= a_n \frac{X(X-1)...(X-n+1)}{n!}+a_{n-1} \frac{X(X-1)+...+(X-n+2)}{(n-1)!}+...\cr &+a_{2} \frac{X(X-1)}{2!}+a_1X+a_0 \,.\cr \end{align} $$

  See if you can prove this!

Week 5

• Problem (posted October 3rd)

  The sequence $a_n$ is defined by $a_1=1$ and for all $n \geq 1$ $$ a_{n+1}=\frac{a_n}{1+n a_n} \,.$$

  Find $a_{1996}$.

• Solution (posted October 10th)

  This is problem 5 of the 1999 Íslenzka Staerŏfræŏikeppni Framhaldsskólanema which appeared in Crux Mathematicorum in [2001:232-233]. We give the solution of Michel Bataille that appeared in Crux Mathematicorum in [2003:304].

  It is easy to see that $a_n >0$ for all $n$. Then, for all $n$ we have $$ \frac{1}{a_{n+1}}=\frac{1}{a_n}+n \,.$$

  Therefore, $$ \begin{align} \frac{1}{a_{1996}}-1 &= \frac{1}{a_{1996}}-\frac{1}{a_1}= \sum_{k=1}^{1995} \left( \frac{1}{a_{k+1}}-\frac{1}{a_k} \right) \cr &= \sum_{k=1}^{1995} k =\frac{1995 \cdot 1996}{2} =1991010 \cr \end{align} $$

  Therefore $$a_{1996}=\frac{1}{1991011} \,.$$

Week 4

• Problem (posted September 26th)

  Find all polynomials $P(X)$ satisfying $$(X-16)P(2X)=16(X-1)P(X) \,.$$

• Solution (posted October 3rd)

  This is problem 3 of the 10th Irish Math Olympiad 1997, which appeared in Crux Mathematicorum in [2001:6-8], with solution appearing in Crux Mathematicorum in [2003:96]. We give an alternate solution.

  Setting $X=16$ in the given equation we get $P(16)=0$ and hence, we can write $P(X)=(X-16)P_1(X)$ for some polynomial $P_1(X)$. The given relation then becomes $$(X-8)P_1(2X)=8(X-1)P_1(X) \,.$$

  Setting $X=8$ we get $P_1(8)=0$ and hence, we can write $P_1(X)=(X-8)P_2(X)$ for some polynomial $P_2(X)$. Thus, we get $$(X-4)P_2(2X)=4(X-1)P_2(X) \,.$$

  Setting $X=4$ we get $P_2(4)=0$ and hence, we can write $P_2(X)=(X-4)P_3(X)$ for some polynomial $P_3(X)$. Thus, we get $$(X-2)P_3(2X)=2(X-1)P_3(X) \,.$$

  Setting $X=2$ we get $P_3(2)=0$ and hence, we can write $P_3(X)=(X-2)P_4(X)$ for some polynomial $P_4(X)$. Thus, we get $$P_4(2X)=P_4(X) \,.$$

  Then, we have $P_4(1)=P_4(2)=P_4(4)=P_4(8)=...=P_4(2^n)=..$ and hence $P_4(X)=a$ for some constant $a$.

  Answer: $P(X)=a(X-16)(X-8)(X-4)(X-2)$.

Week 3

• Problem (posted September 19th)

  This week we look at a point inside a square.

  A point $E$ inside a square $ABCD$ is such that $AE=5, BE=2\sqrt{2}$ and $CE=3$. Determine the area of $ABCD$.

• Solution (posted September 26th)

  This is problem 3 of Alberta High School Math Competition 2002, Part B. We present the solution of Keith Chung from the contest.

  Drop perpendiculars $EF$ and $EG$ from $E$ onto $AB$ and $AC$ respectively.

  

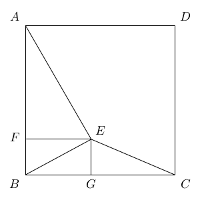
  Denote $$ \begin{align} EF & = x \cr EG & =y \cr AB & =s \,. \end{align} $$

  Then $$ \begin{align} x^2+y^2&=8 \tag{1}\cr (s-x)^2+y^2&=9\tag{2}\cr x^2+(s-y)^2&=25 \tag{3} \end{align} $$

  From (1) and (2) we get $s^2-2sx=1$ and hence $$ x=\frac{s^2-1}{2s} \,. $$

  From (1) and (3) we get $s^2-2sy=17$ and hence $$x=\frac{s^2-17}{2s} \,.$$

  Plugging back in (1) we get $$(s^2-1)^2+(s^2-17)^2=32s^2 \,,$$

  or, equivalently $$(s^2-5)(s^2-29)=0 \,.$$

  Since $E$ is inside $ABCD$ and $AE=5$, we cannot have $s=\sqrt{5}$. Therefore $s^2=29$, so the desired area is 29.

Week 2

• Problem (posted September 12th)

  This week we look at an equation with integer roots.

  Determine all rational numbers $r$ for which all the solutions of the equation $$ rx^2+(r+1)x+r-1=0 $$ are integers.

• Solution (posted September 19th)

  Problem 5 of the $21^{\mbox{st}}$ Austian Mathematical Olympiad, Final Round, 1990, which appeared in Crux Mathematicorum [1992:100]. We present the similar solutions by Joseph Ling, Pavlos Maragoudakis and Michael Selby which appeared at [1993:138].

  If $r=0$ then the equation becomes $x-1=0$, so $x=1$. Therefore $r=0$ is a solution.

  If $r \neq 0$, let then $x_1 \leq x_2$ be the roots of the quadratic equation. Then $$ \begin{align} x_1+x_2 &= -\frac{r+1}{r}=-1-\frac{1}{r} \cr x_1x_2 &= \frac{r-1}{r}=1-\frac{1}{r} \,. \end{align} $$

  Then $$ \begin{align} x_1x_2-(x_1+x_2) &= 2 \cr (x_1-1)(x_2-1) &= 3 \,. \end{align} $$

  Therefore, as $x_1 \leq x_2$ are integers, we either have $x_1=2, x_2=4$ or $x_1=-2, x_2=0$.

  In the first case we get $r=-\frac{1}{7}$ while in the second we get $r=1$.

  Answer: $r \in \{ -\frac{1}{7}, 0, 1 \}$.

Week 1

• Problem (posted September 5th)

  We give two entry level problems this week. Give them a try. Look for the source and the solution next week!

  Problem A
  Prove that the number $$ \underbrace{111...111}_{1997} \underbrace{222...222}_{1998} 5 $$ is a perfect square.

  Problem B
  In the diagram, $ABCD$ is a rectangle with $AD=1$, and both $BF$ and $DE$ are perpendicular to the diagonal $AC$. We further have $AE=EF=FC$. Find the length of the side $AB$.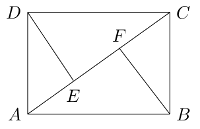

  

• Solution (posted September 12th)

  Problem A

  Problem 1 of the 2^nd Junior Balkan Math Olympiad, 1998, which appeared in the Skoliad Corner of Crux Mathematicorum at [2002:522]. We present the official solution that appeared at [2003:262].

  $$ \begin{align} \underbrace{111...111}_{1997}\underbrace{222...222}_{1998}5 &=\underbrace{111...111}_{1997}\underbrace{000...000}_{1999}+2\cdot \underbrace{111...111}_{1998}0+5 \cr & = \frac{10^{1997}-1}{9}\cdot10^{1999}+2 \cdot \frac{10^{1998}-1}{9}\cdot10+5\cr & =\frac{1}{9} \left(10^{3996}-10^{1999}+2\cdot10^{1999}-20+45 \right) \cr & =\frac{1}{9} \left(10^{3996}+\cdot10^{1999}+25 \right) \cr & =\frac{1}{9} \left(10^{3996}+2 \cdot 5 \cdot10^{1998}+5^2 \right) \cr & =\frac{1}{9} \left(10^{1998}+5 \right)^2 =\left(\frac{10^{1998}+5}{3}\right)^2=\left(\frac{10^{1998}-1}{3}+2\right)^2 \cr &=\left( \underbrace{333...333}_{1998}+2 \right)^2 \end{align} $$

  Problem B

  Problem 10 of the British Columbia Secondary School Mathematics Contest, 2008, Junior Final, Part A which appeared in the Skoliad Corner of Crux Mathematicorum at [2008:321-324]. We present the solution by Jixuan Wang that appeared at [2009:269].

  Let $x$ be the common length of $AE, EF$ and $FC$. Then, $EC=2x$.

  By the Pythagorean Theorem, $AE^2+DE^2=AD^2$ and hence $$ DE=\sqrt{1-x^2} \,. $$

  Note that $$ \angle ECD =90^\circ -\angle EDC =\angle ADE \,, $$ and therefore $$ \Delta DEA \sim \Delta CED \,. $$

  We therefore get $$ \begin{align} \frac{EA}{DE} &=\frac{ED}{CE} \qquad \Rightarrow \cr \frac{x}{\sqrt{1-x^2}} &= \frac{\sqrt{1-x^2}}{2x} \qquad \Rightarrow \cr 2x^2&=1-x^2 \qquad \Rightarrow \cr x&=\frac{1}{\sqrt{3}} \end{align} $$

  Thus, $AC=3x =\sqrt{3}$ and by the Pythagorean Theorem in $\Delta ABC$ we get $$ AB= \sqrt{2} \,. $$