Week 9

• Problem (posted October 28th)

  Time to test our strengths with geometry and perhaps add a new tool to the toolbox. Here you need some basic facts about points, circles and lines. Google "the power of a point".

  A circle $(O,r)$ and a point $A$ outside the circle are given. From $A$ we draw a straight line $\epsilon$, different from the line $AO$, which intersects the circle at $B$ and $\Gamma$, with $B$ between $A$ and $\Gamma$. Next we draw the symmetric line of $\epsilon$ with respect to the axis $AO$, which intersects the circle at $E$ and $\Delta$, with $E$ between $A$ and $\Delta$.

  Prove that the diagonals of the quadrilateral $B \Gamma \Delta E$ pass through a fixed point; that is they always intersect at the same point, independent of the position of the line $\epsilon$.

• Solution (posted November 4th)

  This problem was problem 3 of the Hellenic Mathematical Competitions 2004 which appeared in Crux Mathematicorum [2007:336-337]. The solution given was that of Pavlos Maragoudakis, given in Crux Math. at [2008:288].

  

  Let $AO \cap B\Delta = \{ P \} $. By symmetry, $\angle A\Gamma P = \angle B \Delta A.$ Since $\angle B \Delta P = \frac{1}{2} \angle BOE = \angle BOA,$ we have $\angle A \Gamma P = \angle BOA.$ Thus, triangles $ A \Gamma P$ and $ AOB$ are similar, so $$ \frac{AP}{AB} = \frac{A \Gamma}{AO},$$ or $AP = \frac{AB \circ A \Gamma}{AO}.$

  Since $AB\circ A \Gamma = AO^2 - r^2,$ we have $AP = \frac{AO^2 -r^2}{AO},$ which is independent of the line $\epsilon.$ By symmetry, $E \Gamma$ and $AO$ also intersect at the point $P$, and the proof is complete.

Week 8

• Problem (posted October 21st)

  This week we turn to a type of problem that typically involves an analysis of the situation in terms of smaller versions of the same problem.

  On a $2 \times n$ board, you start from the square at the bottom left corner. You are allowed to move from a square to an adjacent square, with no diagonal moves, and each square must be visited at most once. Moreover, two squares visited on the path may not share a common edge unless you move driectly from one of them to the other. We consider two types of paths, those ending on the square at the top right corner and those ending on the square at the bottom right corner. The diagram below shows that there are $4$ paths of each type when $n = 4.$ Prove that the numbers of these two types of paths are the same for $n = 2014.$

  
• Solution (posted October 28th)

  This was Problem 5 of part 2 of the 2013-2014 Alberta High School Mathematics Competition. We give the "`official"' solution.

  The path of the marker is uniquely determined by its vertical moves. The only condition is that no two vertical moves can be made in adjacent columns. Whether the path ends in the upper or lower right corner is determined by the parity of the number of vertical moves. Let the columns be represented by elements in the set $\{1,2,\ldots,n\}.$ Consider all subsets which do not contain two consecutive numbers. Let $a_n$ be the number of such subsets of even size, and $b_n$ be the number of such subsets of odd size. Then $a_0 = a_1 = a_2 = 1$ because of the empty subset, $b_0 = 0, b_1 =1$ and $b_2 =2.$

  For $n \geq 3$, classify the subsets of $\{1,2,\ldots,n\}$ into two types, those containing $n-1$ and those not containing $n-1$. A subset of the first type cannot contain either $n-2$ or $n.$ Hence the number of such subsets of even size is $b_{n-3}$ and the number of such subsets of odd size is $a_{n-3}.$ The subsets of the second type may be divided into pairs such that in each pair, the two subsets are identical except that one contains $n$ and the other does not. Hence the number of such subsets of even size is equal to the number of such subsets of odd size. It follows that $a_n-b_n=b_{n-3}-a_{n-3}.$ Hence $$a_{3k}-b_{3k} = (-1)^k(a_0-b_0) = (-1)^k,$$ $$a_{3k+1}-b_{3k+1} = (-1)^k (a_1-b_1)=0,$$ $$a_{3k+2}-b_{3k+2} = (-1)^k(a_2 -b_2) = (-1)^{k+1}.$$ In particular, $a_{2014} = b_{2014}.$

Week 7

• Problem (posted October 14th)

  This week a geometry problem that only calls for basic facts about circles and some use of trigonometry.

  Let $k$ be a circle with centre $O$ and let $AB$ be a chord of $k$ whose midpoint $M$ is distinct from $O.$ The ray from $O$ through $M$ meets $k$ again at $R.$ Let $P$ be a point on the minor arc $AR$ of $k$, let $PM$ meet $k$ again at $Q$, and let $AB$ meet $QR$ at $S.$ Which segment is longer, $RS$ or $PM?$

• Solution (posted October 21st)

  This was problem 5 of the Hungarian Mathematical Olympiad 2005-2006 Specialized Mathematical Classes, First Round, given in Crux Mathematicorum at [2009:212]. The solution by Geoffrey Kandall, Connecticut USA was given at Crux [2010:282].

  Extend $RO$ to meet $k$ at $T.$ Note that $\angle RMS, \angle RQT$ are right angles.

  Let $\angle MRP = \theta, \angle MRS = \phi.$ Then $RM = RS \cos \phi$ and $ \angle RPQ = \angle RTQ = 90^\circ - \phi.$ Consequently, $$ \frac{PM}{\sin \theta} = \frac{RM}{\sin (90^\circ - \phi)} = \frac{RM}{\cos \phi } = RS ,$$ that is $PM = RS \sin \theta < RS.$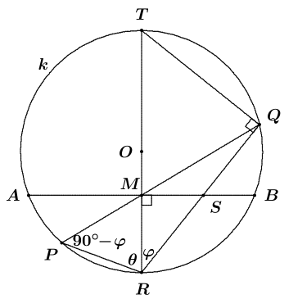

  

Week 6

• Problem (posted October 7th)

  It is time to exercise our skills with inequalities.

  Let $p,q,$ and $r$ be positive real numbers and let $n$ be a positive integer. If $pqr = 1$, prove that $$ \frac{1}{p^n+q^n+1} + \frac{1}{q^n + r^n +1} + \frac{1}{r^n + p^n +1} \leq 1.$$

• Solution (posted October 14th)

  This was problem 3 of the Mathematical Competition Baltic Way 2004 given in Crux Mathematicorum at [2008:211-213]. The solution by Titu Zvonaru, Romania, appeared in Crux at [2009:150-151].

  Let $p^n = x^3, q^n= y^3,$ and $r^n = z^3.$ We must prove that if $xyz =1$ then $$ \frac{1}{x^3+y^3+1} + \frac{1}{y^3+z^3+1} +\frac{1}{z^3 + x^3 +1} \leq 1,$$ or equivalently we must prove that $$ \frac{1}{x^3 + y^3 + xyz} + \frac{1}{y^3 + z^3 + xyz } + \frac{1}{z^3 +x^3 +xyz} \leq 1.$$

  Since $x$ and $y$ are positive, $(x-y)^2(x+y) \geq 0$, hence $(x-y)(x^2-y^2) \geq 0,$ hence $x^3 + y^3 \geq xy(x+y).$ It follows that

  ${\large \begin{array}{llllll} & \frac{1}{x^3+y^3+xyz} & + & \frac{1}{y^3+z^3+xyz} & + & \frac{1}{z^3+y^3 +xyz} \newline \leq & \frac{1}{xy(x+y) +xyz} & + & \frac{1}{yz(y+z) +xyz} & + & \frac{1}{zx(z+x) + xyz} \newline = & \frac{x+y+z}{xyz(x+y+z)} & = & 1. & & \end{array} }$

  Equality holds if and only if $x=y=z=1$, or if and only if $p=q=r=1.$

Week 5

• Problem (posted September 30th)

  Next a problem involving counting and sums.

  In how many ways can the number $2000$ be written as a sum of three positive, not necessarily different, integers? (Sums like $1+2+3$ and $3 +2 +1$ are considered to be the same.)

• Solution (posted October 7th)

  This was problem 1 of the Nordic Mathematical contest and appeared in Crux Mathematicorum at [2003:435]. The solution is by Michel Bataille of Rouen, France. It appeared in Crux Mathematicorum at [2004:381].

  We are required to find the number of triples $(n_1,n_2,n_3)$ of integers such that $ 1 \leq n_1 \leq n_2 \leq n_3$ and $n_1 + n_2 + n_3 = 2000.$ This number is 333333, the nearest integer to $2000^2/12$, as we shall now prove.

  More generally, let $n$ be any integer with $n\geq 3$, and let $t_n$ be the number of triples $(n_1,n_2,n_3)$ with $1 \leq n_1 \leq n_2 \leq n_3$ and $n_1+n_2+n_3 = n$. For $n \geq 2$, we similarly denote by $d_n$ the number of pairs $(n_1,n_2)$ such that $1 \leq n_1 \leq n_2$ and $n_1 + n_2 =n.$

  Clearly, $d_2 = d_3 = 1,$ and more generally, it is readily seen that $d_n = n/2$ if $n$ is even and $d_n = (n-1)/2$ if $n$ is odd. Returning to $t_n$, we find that $t_3 = t_4 = 1$ and $t_5 =2.$ For $n \geq 6$, we have $t_n = d_{n-1} +t_{n-3}$, because there are $d_{n-1}$ triples for which $n_1 =1$, and the set of suitable triples with $n_1 \geq 2$ is obviously in bijection with the set of triples $(n_1-1,n_2-1,n_3-1)$ summing to $n-3.$

  Now, assume that $n = 6k +2$ for some positive integer $k$. Then $$\begin{array}{ccc} t_n & = & (t_{6k+2}-t_{6k-1})+ (t_{6k-1} -t_{6k-4}) + \cdots \newline & & +(t_{11}-t_8) + (t_8 -t_5) +t_5 \newline & = & d_{6k+1} + d_{6k-2} + d_{6k-5} + \cdots + d_7 + d_5 \newline & = & 3k +(3k-1) + (3k -3) + (3k-4) + \cdots + 6 + 5 + 3 + 2 \newline & = & \frac{k(3k+1)}{2} + \frac{k(3k+1)}{2} = k(3k+2) = \frac{n^2}{12} - \frac{1}{3}, \end{array}$$

  which is the integer nearest to $n^2/12.$ For $n=2000$ this gives $t_n = 333333.$ (Examining the cases $n=6k, 6k+1,6k+3, 6k+4, 6k+5$ in the same way, it is easy to show that $t_n$ is always the integer nearest to $n^2/12.$)

Week 4

• Problem (posted September 23rd)

  This week we look at a type of problem called a functional equation.

  Find all functions $f : \mathbb {R} \rightarrow {\mathbb R}$ such that $$ f(xf(x) + f(y)) = x^2 +y$$ for all $x \in \mathbb {R}$ and $y \in \mathbb{R}.$

• Solution (posted September 30th)

  This was problem 6 of the Ukraine Mathematical Olympiad 11th Form given in Crux Mathematicorum [2006:217-218]. The solution by Li Zhou of Polk Community College appeared in Crux Mathematicorum [2007:228].

  It is easy to verify that both $f(x) = x$ and $f(x) = -x$ satisfy the functional equation. We will prove that these are the only solutions.

  Let $f$ be a solution. Setting $x=0$ in the functional equation gives $f(f(y)) = y$ for all $y \in \mathbb {R}.$ Hence, for all $x, y \in \mathbb {R},$ $$x^2+y = f(xf(x)+f(y)) = f(f(x)f(f(x)) + f(y)) = f(x)^2 +y.$$ Thus, $f(x)^2 = x^2$ for all $x \in \mathbb{R}$. If $f(1)=1$, then $$ 1+2x+x^2 = f(1+x)^2 =f(1f(1) + f(f(x)))^2$$ $$ = (1^2 +f(x))^2 = 1 +2f(x) +x^2,$$ and thus $f(x) = x$ for all $x \in \mathbb{R}.$

  Similarly, if $f(1)= -1$, then $$ 1-2x+x^2 = f(-1+x)^2 = f(1f(1) + f(f(x)))^2$$ $$ = (1^2 +f(x))^2 = 1 + 2f(x) +x^2,$$ and thus $f(x) = -x$ for all $ x \in \mathbb{R}.$

Week 3

• Problem (posted September 16th)

  This week we look at a problem which draws on basic algebra from high-school.

  Let $x,y,$ and $z$ be real numbers such that $\begin{array}{ccc} x+y+z =3 & \mbox{and} & xy + yz + xz = a \end{array}$, where $a$ is a real parameter. Determine the value of the parameter $a$ for which the difference between the maximum and the minimum possible values of $x$ equals $8.$

• Solution (posted September 23rd)

  This problem was problem 1 of the 7th National Olympiad of Bosnia and Herzegovina 2002, given in Crux Mathematicorum at [2005:436]. The solution is by Pierre Bornsztein of Maisons-Laffitte, France. It appeared in Crux at [2007:22].

  We have $y+z = 3-x$ and $yz = a -x(3-x).$ It is easy to verify that the system $$\begin{array}{ccc} y+z & = & s \newline yz & = & p \end{array}$$ with unknowns $y$ and $z$, has a real solution if and only if $s^2 -4p \geq 0.$

  Hence, the unique condition we have to satisfy for there to be real solutions is $(3-x)^2 \geq 4(a-3x + x^2),$ or $3(x-1)^2 \leq 4(3-a).$ That is, $a\leq 3$ and $$1-2 \sqrt{1 - \frac{1}{3}a} \leq x \leq 1 + 2 \sqrt{1 \ \frac{1}{3}a}.$$

  The difference betwwen the maximum and minimum possible values of $x$ equals $8$ if and only if $4\sqrt{1-\frac{1}{3}a} = 8,$ which implies that $a = -9.$

Week 2

• Problem (posted September 9th)

  The problem for this week is a problem involving velocities. It takes some thought to find how to get started!

  Two cars 100 metres apart are traveling in the same direction along a highway at the speed limit of 60 km/h. At one point on the highway, the speed limit increases to 80 km/h. Then a little later, it increases to 100 km/h. Still later, it increases to 120 km/h. Whenever a car passes a point where the speed limit increases, it instantaneously increases its speed to the new speed limit. When both cars are traveling at 120 km/h, how far apart are they?

• Solution (posted September 16th)

  This was problem 3 of Part 2 of the 2013-2014 Alberta High School Prize Examination.

  Let the first car be at a point $B$ while the second car is at a point $A$ , both in the 60 km/h zone. Then $AB = 100$ meters. Let the first car be at a point $D$ while the second car is at a point $C$, both in the 120 km/h zone. Now the amount of time the second car takes to go from $A$ to $C$ is the same as the amount of time the first car takes to go from $B$ to $D$. Both cars take the same amount of time going from $B$ to $C$ . Hence the amount of time the second car takes to go from $A$ to $B$ at 60 kph is the same as the amount of time the first car takes to go from $C$ to $D$ at 120 kph. It follows that $CD = 2AB = 200$ meters.

Week 1

• Problem (posted September 2nd)

  For the first week we look at two problems. The first does not require work to be shown, and is a puzzle problem. The second requires work to be shown. It involves digits of numbers and divisibility. Look next week to check your solution.

  Problem A
  A home has some fish, some birds and some cats. Altogether there are 15 heads and 14 legs. If the home has more than one of each animal, how many fish are there?

  Problem B
  There are 2014 digits in a row. Any two consecutive digits form a number which is divisible by 17 or 23.

  1. If the last digit is 1, then what are the possibilities for the first digit?
  2. If the first digit is 9, then what are the possibilities for the last digit?
• Solution (posted September 9th)

  Problem A
  [This problem was A6 from the 2014 Calgary Junior Mathematics Contest. ]
  Let there be $F$ fish, $B$ birds and $C$ cats. From the legs we get $2B +4C =14$, so $B + 2C =7$ and from the heads $F+B+C = 15$. Now there are more than one of each type, so there are at least 2 birds. But then there can be at most 2 cats, so $C=2$ and $B=3$. From this we get $F = 15-2-3 =10.$

  Problem B
  [This problem was B2 from the 2014 Calgary Junior Mathematics Contest. ]
  

  1. The two digit multiples of $17$ are $17,\ 34, \ 51, \ 68$ and $85.$ Those of $23$ are $23,\ 46,\ 69$ and $92.$ We now can work backward from right to left working two digits at a time starting with $x1$. This gives $51$, then with $x5$ we get $851$, then $6851$, then $46851$, $346851$, $2346851$, $92346851$, and next $692346851$. It is now clear that the pattern $92346$ of length five will repeat. Now $2014 = 402 \times 5 + 4$, so the number must be $402$ blocks of $69234$ followed by $6851$, so the first digit is $6.$
  2. With the same method as before but starting with $9y$ we get $92$, then $923$, then $9234$ and then $92346$. At this point we have both a multiple of $17$, namely $68$ and a multiple of $23$, namely $69$ to consider. Let us first pursue the path with $68$, i.e. continuing to $923468$, then $9234685$, and $92346851$ and next $923468517$, where we are stuck since we cannot have $7y$ appearing. What happens with the possibility from $69?$ Here we observe, as in the first example that a cycle $92346$ of length $5$ is formed. Now we see that two possible numbers could be formed. One is $402$ blocks of $92346$ ended by $8517$ and the other is $402$ blocks of $92346$ ended by $9234$. So the two possibilities for the last digit are $4$ and $7.$