La SMC organisera des mini-cours de trois heures pour accroître l’attrait de ses Réunions et inciter plus d’étudiants et de chercheurs à y assister.
Les mini-cours auront lieu le vendredi 4 decembre en après-midi, avant la conférence publique, et porteront sur des sujets adaptés aux étudiants des cycles supérieurs, aux postdoctorants ou à toute personne intéressée. Vous pouvez vous inscrire aux mini-cours sans être inscrit.e a la réunion.
Les frais d’inscription aux mini-cours sont les suivants :
Tarif normal |
|||
| Étudiants/Postdoctorants (membres) | $50 |
||
| Étudiants/Postdoctorants (non-membres) | $75 |
||
| Membres de la SMC | $100 |
||
| Non-membres de la SMC | $150 |
Mini-cours
An Introduction to Programming in Maple

Thursday, December 3rd
11:00- 14:00
Complimentary Mini Course
Facilitator: Paulina Chin, Software Architect, Maplesoft
Abstract
In addition to being an interactive environment for problem-solving, visualization, and technical document preparation, Maple also features a powerful programming language that is especially useful for working with mathematics. Becoming familiar with the Maple language will allow you to increase the range and efficiency of what you can do in Maple, from writing short scripts to automating a repetitive calculation, to creating interactive applications for students and developing new algorithms to advance your research.
This course will begin with an overview of the Maple software package, highlighting a number of its important features. We will then cover the basics of the Maple language, common data structures, and writing simple programs. We will also look briefly at tools to aid the construction of larger programs and packages and the building of interactive applications in the style of Maple’s Math Apps.
Newcomers to Maple, as well as experienced users who would like to learn more about the Maple language, are welcome.
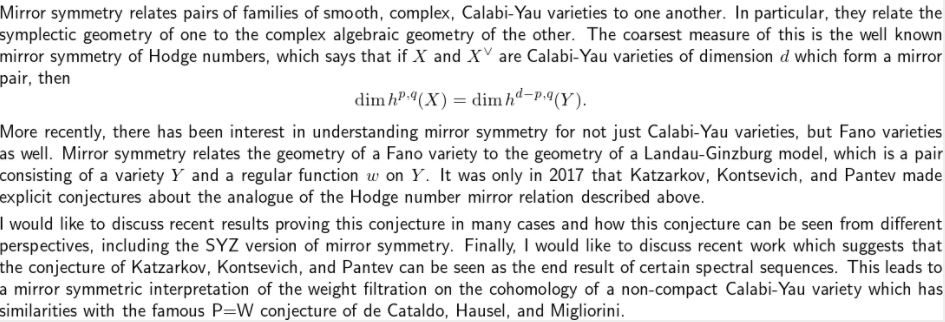
Filtrations on cohomology in mirror symmetry
Thursday, December 3rd
Facilitator: Andrew Harder (Lehigh University)
Moderator: Charles Doran (Harvard)
Abstract
Symplectic Reduction and Geometric Invariant Theory
Thursday December 3rd
14:30-17:30pm
Facilitators: Michael Groechenig (University of Toronto) and Steven Rayan (University of Saskatchewan)
Speaker: Maxence Mayrand (University of Toronto)
Abstract
There is a rich history of interactions between symplectic and complex geometry. One important aspect of it, Kempf-Ness type theorems, link beautifully the two natural notions “quotient spaces” in these geometries. On the one hand, symplectic reduction, which has roots in classical mechanics, can be performed when a real Lie group acts on a smooth symplectic manifold, and a choice of moment map has been made. On the other hand, Geometric Invariant Theory, which is the main tool for moduli problems in algebraic geometry, produces quotients of complex algebraic varieties by complex Lie groups upon choosing a line bundle with a lift of the action. The natural ground where both structures coexist is Kähler geometry, and in that case, there is a correspondence between these two choices so that the quotients coincide. This mini-course aims to present this result in the general context of non-compact and singular spaces, as well as in hyperkähler geometry, and to illustrate it by many examples, both finite and infinite-dimensional.
Student Committee Mini-Course
Thursday, December 3rd
14:30-17:30pm
Speakers: Mike Bennett (University of British Columbia), Stacey Smith? (University of Ottawa), and NSERC.
Abstract
The student committee of the CMS has prepared a workshop on communication in mathematics. We will hear about how to write a good paper (with mathematical modelling as an example), how to write good scholarships and fellowships applications, and how the recent pandemic has influenced communication in mathematics.
The Lovász Local Lemma and its applications in ergodic theory and descriptive set theory
Thursday, December 3rd
14:30 - 17:30
Facilitator: Marcin Sabok (McGill) and Anush Tserunyan (McGill)
Speaker: Anton Bernshteyn (Georgia Tech)
Abstract
The Lovász Local Lemma (the LLL, for short) is a powerful probabilistic result that was introduced by Erdős and Lovász in 1975 and has since become an indispensable tool in combinatorics and graph theory. The LLL is mostly used to obtain existence results, and it is particularly well-suited for showing that a given structure admits a partition or a “coloring” satisfying some “local” constraints. It has recently transpired that, in addition to its applications in combinatorics, the LLL can also be quite useful in descriptive set theory and in the study of dynamical systems. The aim of this mini-course is to provide an introductory guide to the LLL for those interested in applying it in these areas.
